Light travels at 299,792,458 meters per second. That’s not so hard to think about, it’s just a really quick-moving item. This simple answer is easily muddied when you ask yourself, “What is moving at that speed?”

When I first looked at this problem, I thought that light travelled up and down a wave, like the iPhone game Tinywings, and the light speed could be found by grabbing the ends of that wave and pulling them taut. Light is a particle and a wave, right? So I imagined it as a surfer.

In fact, though, a wave’s speed is the amount of time it takes for the crest of the wave itself to move from one point to another. And if we want to understand lightspeed, light as a wave is the best way to do it.

Waves are sometimes called “propagations of disturbance.” I like the phrase’s poetic ring, but it also explains what waves really are. In a wave, nothing actually moves. This may sound impossible, but it is true. Take a look at this:

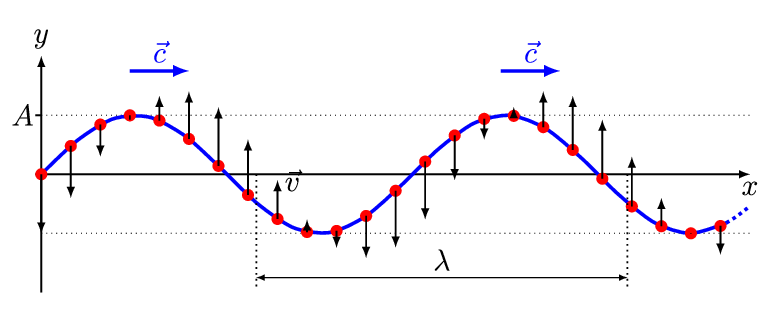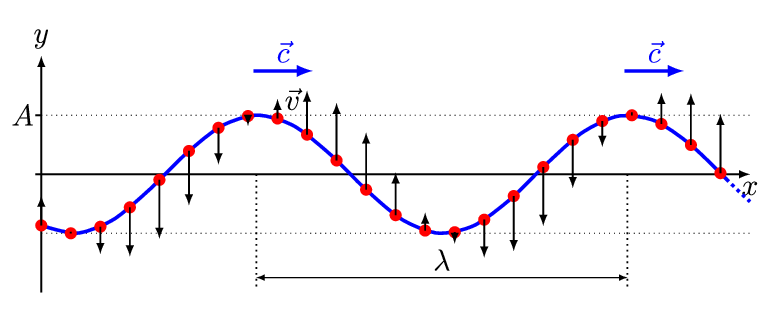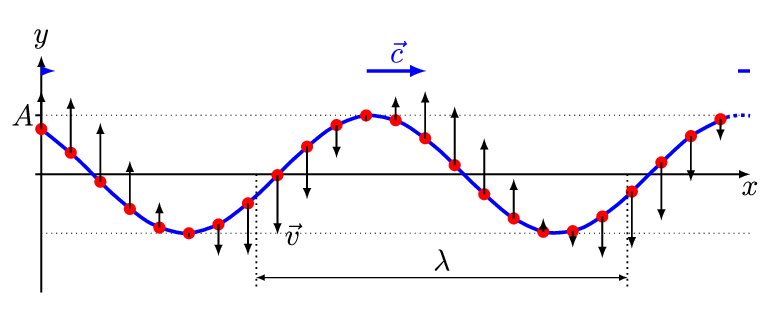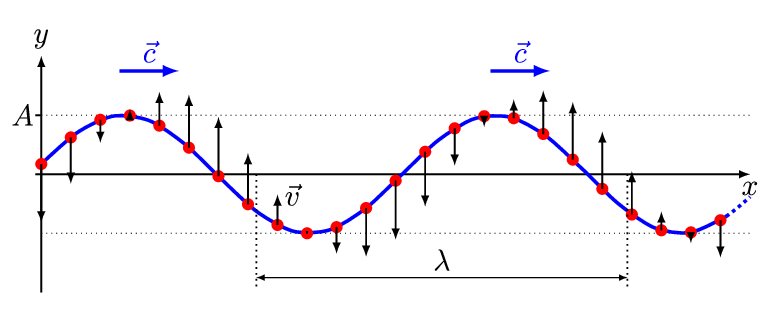
It’s a wave right? And it’s moving at the speed of the blue arrow. But if you look at any individual point, it is only moving up and down. In a water wave, for example, a moving particle pulls down the one next to it through a force (in water it is hydrogen dipole-dipole forces). It builds up some speed, and then is slowly dragged to a stop by it’s neighbor changing direction, restarting the cycle. In light, however, there is no force, there is just a wave. We’re stuck.

Actually, it turns out to be a good thing that there are no individual items moving within the light. This way, we can model it as a function. If you look at the wave again, you can see a blue line that interpolates between all the points. Since light acts as a continuous function, you can use that wave, a sine wave, to think about the propagation of light, with each x value on the infinitely dense numberline moving up and down to the rise an fall of the sine function like the bows of an orchestra to the baton of the conductor.

Since light moves through both time and space, we can put time and space as the inputs and height as the output.

Let’s take a tour of the orchestra. ‘A’ stands for amplitude, the amount of maximum distance that the wave moves up and down in a given amount of time. Though amplitude determines the strength of light, it does not determine the distance the light travels. What we are really worried about is what defines the distance between the crests. This value is defined by T and L. Big T represents the "temporal pattern." The temporal pattern how long it takes for the wave to go up and down again. The bigger the T, the more often it goes up and down. Big L (sometimes called lambda) is the "spacial pattern." It determines the distance between each peak. The smaller the L, the less it goes up and down (oscillates).

If L is space and T is time, then L divided by T is space divided by time, i.e. meters per second. As it turns out, L / T is always 299,792,458! This is what light speed it. It is the fact that the ratio of time to space in light waves is held constant. But what does that mean?

c = λ/ν

Imagine you are resizing an image in powerpoint and you don’t want it to get squashed, so you hold shift and the dimensions are preserved. If you imagine a square being drawn where one corner is at the crest of the wave and the other corner is at the trough before it, light acts the opposite. Instead of preserving dimensions like in powerpoint; in light, area is preserved. This means T gets shorter when L gets longer and skinnier when L gets taller. In the graph, this shows as the peaks of every wave being on the same line no matter what. You can move that line around when you change lightspeed or ‘c’.

If you fidget L around, you are traveling through the electromagnetic spectrum. The bigger it is the bigger the wavelength. Here’s something to ponder: only some wavelengths can travel through air.

All of light, radio, Wi-Fi, cell towers, and TV has to fit within those holes. Planet money has a great podcast on the business challenges of selling off those holes.

So godspeed and lightspeed, more on communications another time!